How To Add Fractions With Different Denominators With Variables
Now let's acquire how to add together fractions with different denominators. When fractions are added, the denominators of those fractions should exist the same. But they are not always the same.
For example, fractions ![]() and
and ![]() tin can exist added together because they have the same denominators.
tin can exist added together because they have the same denominators.
But fractions ![]() and
and ![]() cannot be added together at in one case because these fractions have different denominators. In such cases, fractions must be reduced to the same (common) denominator.
cannot be added together at in one case because these fractions have different denominators. In such cases, fractions must be reduced to the same (common) denominator.
At that place are several ways to reduce fractions to the same denominator. Today we volition look at just one of them, since the others may seem complicated to a beginner.
The essence of this method is that outset we look for the to the lowest degree mutual multiple (LCM) of the denominators of both fractions. Then the LCM is divided by the denominator of the start fraction and the first additional multiplier is obtained. Do the same with the second fraction - LCM divided past the denominator of the second fraction and get the 2nd boosted multiplier.
And so the numerators and denominators of the fractions are multiplied by their additional multipliers. As a consequence, fractions with different denominators are converted to fractions with the aforementioned denominators. And we already know how to add such fractions.
Example 1. Add fractions ![]() and
and ![]()
These fractions accept different denominators, so you demand to reduce them to the same (mutual) denominator.
Start, find the to the lowest degree common multiple of the denominators of both fractions. The denominator of the kickoff fraction is number 3, and the denominator of the 2d fraction is number 2. The least mutual multiple of these numbers is half dozen
LCM (2 and 3) = half-dozen
Now back to fractions ![]() and
and ![]() . Showtime, dissever the LCM by the denominator of the outset fraction and become the first additional multiplier. The LCM is half-dozen, and the denominator of the first fraction is 3. Dividing 6 by iii, we become ii.
. Showtime, dissever the LCM by the denominator of the outset fraction and become the first additional multiplier. The LCM is half-dozen, and the denominator of the first fraction is 3. Dividing 6 by iii, we become ii.
The resulting number 2 is the first additional multiplier. Write it to the first fraction. To do this, make a pocket-size diagonal line in a higher place the fraction and write the additional multiplier found above information technology:
![]()
Exercise the same with the 2nd fraction. We split the LCM by the denominator of the 2nd fraction and get the second additional gene. LCM is 6, and the denominator of the 2nd fraction is two. Dividing 6 by 2, we go 3.
The resulting number 3 is the second additional multiplier. Write it to the 2d fraction. Again brand a minor diagonal line over the second fraction and write the found additional multiplier over it:
![]()
Now we are all gear up for improver. It remains to multiply the numerators and denominators of the fractions by their additional multipliers:
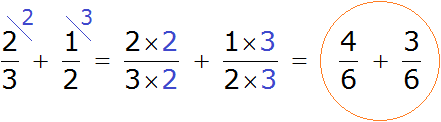
Wait advisedly at what we have come up to. We came to the fact that fractions with different denominators turned into fractions with the same denominators. And nosotros already know how to add together such fractions. Let's solve this example to the finish:
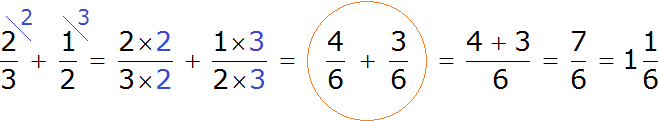
This completes the example. Add ![]() to
to ![]() and you get
and you get ![]() .
.
Let'south try to correspond our solution with a picture. If you lot add ![]() pizza to
pizza to ![]() pizza, you go one whole pizza and ane sixth of a pizza:
pizza, you go one whole pizza and ane sixth of a pizza:
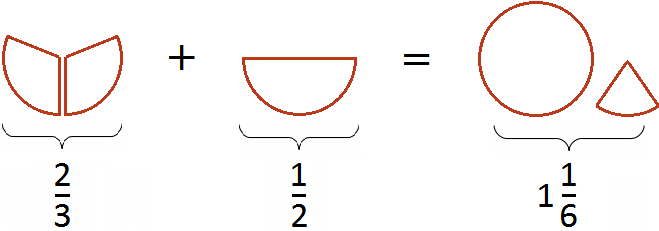
The reduction of the fraction to the same (common) denominator can also be shown. Bringing fractions ![]() and
and ![]() to a common denominator, we got fractions
to a common denominator, we got fractions ![]() and
and ![]() . The two fractions will be represented past the same pieces of pizza. The only difference volition be that this fourth dimension they will be divided into the aforementioned fractions (reduced to the aforementioned denominator).
. The two fractions will be represented past the same pieces of pizza. The only difference volition be that this fourth dimension they will be divided into the aforementioned fractions (reduced to the aforementioned denominator).
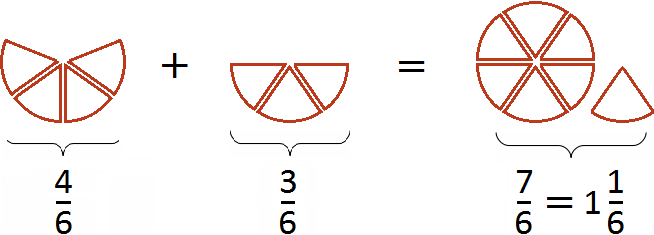
The outset moving picture represents the fraction ![]() (four pieces of six), and the second moving picture represents the fraction
(four pieces of six), and the second moving picture represents the fraction ![]() (three pieces of six). Adding upwardly these pieces we get
(three pieces of six). Adding upwardly these pieces we get ![]() (seven pieces of six). This fraction is improper, and then we highlighted the integer office of information technology. The outcome was
(seven pieces of six). This fraction is improper, and then we highlighted the integer office of information technology. The outcome was ![]() (ane whole pizza and another sixth of a pizza).
(ane whole pizza and another sixth of a pizza).
Note that we have written this example in too much detail. In schools it is not customary to write in such a detailed way. Y'all demand to be able to apace find the LCM of both denominators and additional multipliers to them, and quickly multiply the institute additional multipliers by their numerators and denominators. If we were in school, we would take to write this example as follows:
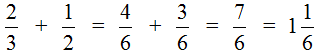
Just there is also the other side of the money. If you don't take detailed notes during the beginning stages of learning math, you get-go to get questions like "where did that number over at that place come from?", "why exercise fractions suddenly turn into completely different fractions?"
Therefore, at the start stages, it is appropriate to write down every detail. Y'all tin can brag only in the hereafter, when the basics have been mastered.
To get in easier to add fractions with different denominators, you tin use the following footstep-by-step instructions:
- Find the LCM of the denominators of fractions;
- Divide the LCM by the denominator of each fraction and obtain an additional multiplier for each fraction;
- Multiply the numerators and denominators of fractions by their boosted multipliers;
- Add fractions that have the aforementioned denominators;
- If the answer is an improper fraction, select the integer function of it;
Instance 2. Find the value of the expression 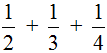 .
.
Let's use the instructions above.
Pace 1. Detect the LCM of the denominators of fractions
Find the LCM of the denominators of both fractions. The denominators of the fractions are numbers 2, 3, and 4.
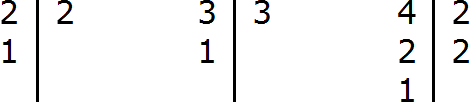
![]()
Step 2: Carve up the LCM past the denominator of each fraction and obtain an boosted multiplier for each fraction
Divide the LCM by the denominator of the first fraction. LCM is 12, and the denominator of the beginning fraction is 2. Carve up 12 by 2 to get 6. Nosotros go the commencement boosted factor 6. Write it over the kickoff fraction:
![]()
At present divide the LCM past the denominator of the second fraction. LCM is 12, and the denominator of the second fraction is 3. We divide 12 by three and get four. Nosotros have a second additional factor 4. Write it over the second fraction:
![]()
Now divide the LCM by the denominator of the third fraction. LCM is 12, and the denominator of the third fraction is four. Nosotros divide 12 by 4 and get 3. We have a third additional factor of 3. Write it over the third fraction:
![]()
Footstep 3: Multiply the numerators and denominators of fractions by their boosted multipliers
Multiply the numerators and denominators by their additional multipliers:
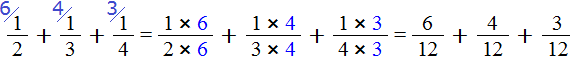
Step 4: Add together fractions with the same denominators
We institute that fractions with different denominators turned into fractions with the same (common) denominators. It remains to add these fractions. We add them up:
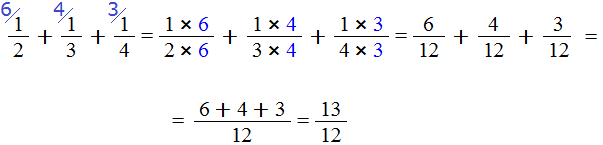
The improver did not fit on one line, so we moved the remaining expression to the next line. This is allowed in mathematics. When an expression does not fit on ane line, information technology is moved to the adjacent line, and you must put an equal sign (=) at the end of the first line and at the starting time of the new line. The equality sign on the second line indicates that it is a continuation of the expression that was on the first line.
Step v. If the respond is an Improper fraction, select the integer part of it
Our answer is an improper fraction. Nosotros must isolate the integer part of it. We do:
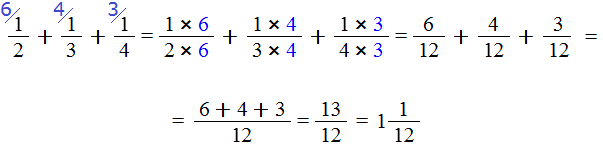
Nosotros got the answer ![]()
Exercises:
Task 1. Find the value of the expression:
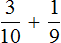
Solution:
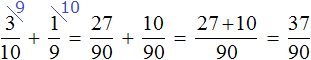
Task ii. Find the value of the expression:
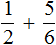
Solution:
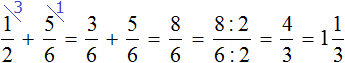
Video lesson
How To Add Fractions With Different Denominators With Variables,
Source: https://math-from-scratch.com/faq/112-adding-fractions-with-different-denominators
Posted by: puckettsectirepas.blogspot.com


0 Response to "How To Add Fractions With Different Denominators With Variables"
Post a Comment